Stellar Formation

Ay 20 – The Formation of Stars

Problem 5

Primary author:  Joanna Robaszewski

Secondary authors:  Cassi Lochhaas and Daniel Lo

Abstract

This problem investigates the initial mass function that regulates mass distribution in star forming regions.  It looks at the number of stars that fall into certain mass ranges and how much each mass group contributes to the overall luminosity and color of the star forming region.

Introduction

The initial mass function describes the distribution of stellar mass at the birth of the star.  It takes on the form:

N is the number of stars that are forming, M is the mass, and A is a proportionality constant to be determined in the problem.  We will be dividing the stars into three groups based on mass.  One will be the low mass stars with a mass range of 0.1M_⊙ to 1M_⊙.  Another will be the intermediate mass group with a range of 1M_⊙ to 8M_⊙.  The last group will contain the high mass stars which fit into the 8M_⊙ and greater range.  M_⊙ is one solar mass and is equal to  2 * 10³³ grams.  Luminosity is a measure of a star’s power output.  The sun has a luminosity of 4 * 10³³ ergs/s.

Questions and Results

5.a  Consider a newly formed globular cluster with total mass of 10⁶ M_⊙ and the initial mass function in the mass range 0.1M_⊙ to 20M_⊙.  Find the proportionality constant A.

We can think of dN/dM as being somewhat similar to a probability density.  From it, we can determine how likely it is that a star forming in this cluster will have a certain mass.  To find the total number of stars in some mass range we use the integral:

To find the total mass of all the stars forming for some mass range, we use the following integral:

In this case we know the total mass of the cluster is 10⁶M_⊙ and the mass range is 0.1 M_⊙ to 20 M_⊙.  So let’s set up the integral:

So we found the proportionality constant A.  If anybody has any ideas as to what the units mean, I’d be happy to hear them.

5.b  What is the fractional number of stars that are fit in the high mass category, the intermediate mass category, and the low mass category (where the categories are defined in the introduction)?  How much mass is contained in each of these mass bins?

We can use the integrals we found in the first part to find the number and mass of the stars in the various mass bins.  All we have to do is pick appropriate boundaries for the mass.

For the high-mass category:

For the intermediate-mass stars:

For the low-mass stars:

So in total we have 3.08 * 10¹⁶ stars in the cluster.  0.19% of these stars are in the high-mass range.  The intermediate-mass range contains 4.2% of the stars in the cluster.  And the low-mass category has 95.5% of the stars in the cluster.  The total mass in the high-mass bin is 1.41*10⁴⁸ grams.  The total mass of all the stars in the intermediate-mass range is 5.49*10⁴⁸ grams.  The total mass found in the low-mass range is 1.31*10⁴⁹ grams.

5.c  The luminosity of a star scales with mass differently for different mass ranges.  The scaling relationships are below:

Low-Mass Stars:  L = M⁵

Intermediate-Mass Stars:  L = M³

High-Mass Stars:  L = 64M

What is the total luminosity of the cluster?  What are the fractional contributions to the total luminosity from each mass range?

First, let’s calculate the luminosity from the low-mass stars.  We can use the same integrals we used to find total mass, but we have to make some substitutions.  An integral for total luminosity would look like this:

So for the low-mass stars:

We also want to convert the boundaries from 0.1 M_⊙ and 1 M_⊙ to (0.1 M_⊙)⁵ and (1 M_⊙)⁵.

The total luminosity for low-mass stars is:

For those of you paying attention, you’ll notice that the units aren’t correct for luminosity.  Ok, so I lied a bit when I said we were calculating the total luminosity contributed by the low-mass stars.  What we actually have is proportionally how much the low-mass stars will contribute to the overall luminosity of the cluster.  Once we calculate the luminosity contributions from the other mass ranges we can find the total contributions toward the cluster’s luminosity.  After that we can find the actual percentages that each mass category contributes to the total luminosity.  So we don’t need to calculate the actual luminosity.  We can just find the proportions based on how luminosity scales with mass for various ranges of mass.

Now we need to calculate the luminosity contribution from the intermediate-mass stars.  The new integral boundaries are now 1M_⊙ and 8M_⊙. 

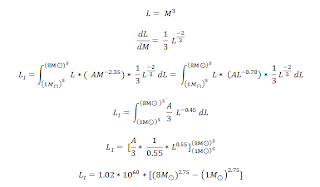
Now to calculate the luminosity contribution from the high-mass stars with integral boundaries 8M_⊙ and 20M_⊙:

Unfortunately these numbers are very far from those that we calculated during class, but I’ve already spent a week and a half trying to get the calculations to work out so I’m just posting what I have.  Please let me know what I’ve left out in my calculations if you figure it out.  In class, my group did the calculations and found a very interesting result.  Even though the stars in the high-mass category were extraordinarily few in number in comparison to other types of stars, they contributed about 55% of the luminosity.  The intermediate-mass stars contributed about 45%, while the low-mass stars contributed less than 1% of the luminosity.  We were all quite surprised and intrigued by this result.

5.d  Would you expect and active star forming galaxy to look more blue or more red?  What about a very old, inactive, elliptical galaxy?

As was stated in the previous section, the high-mass stars contribute the most to the luminosity of the cluster, despite hardly contributing to the number of total stars.  The larger stars that form are blue, while the smaller stars are red.  So since the largest stars are contributing the most to the luminosity of star-forming region, an active star forming galaxy would look blue.  The largest stars are also the ones the die off first, leaving behind the smaller, red stars.  So in an old, inactive galaxy, all the large blue stars are gone.  This leaves the small red stars to contribute most to the luminosity of the galaxy, thereby making it look red.